Answers
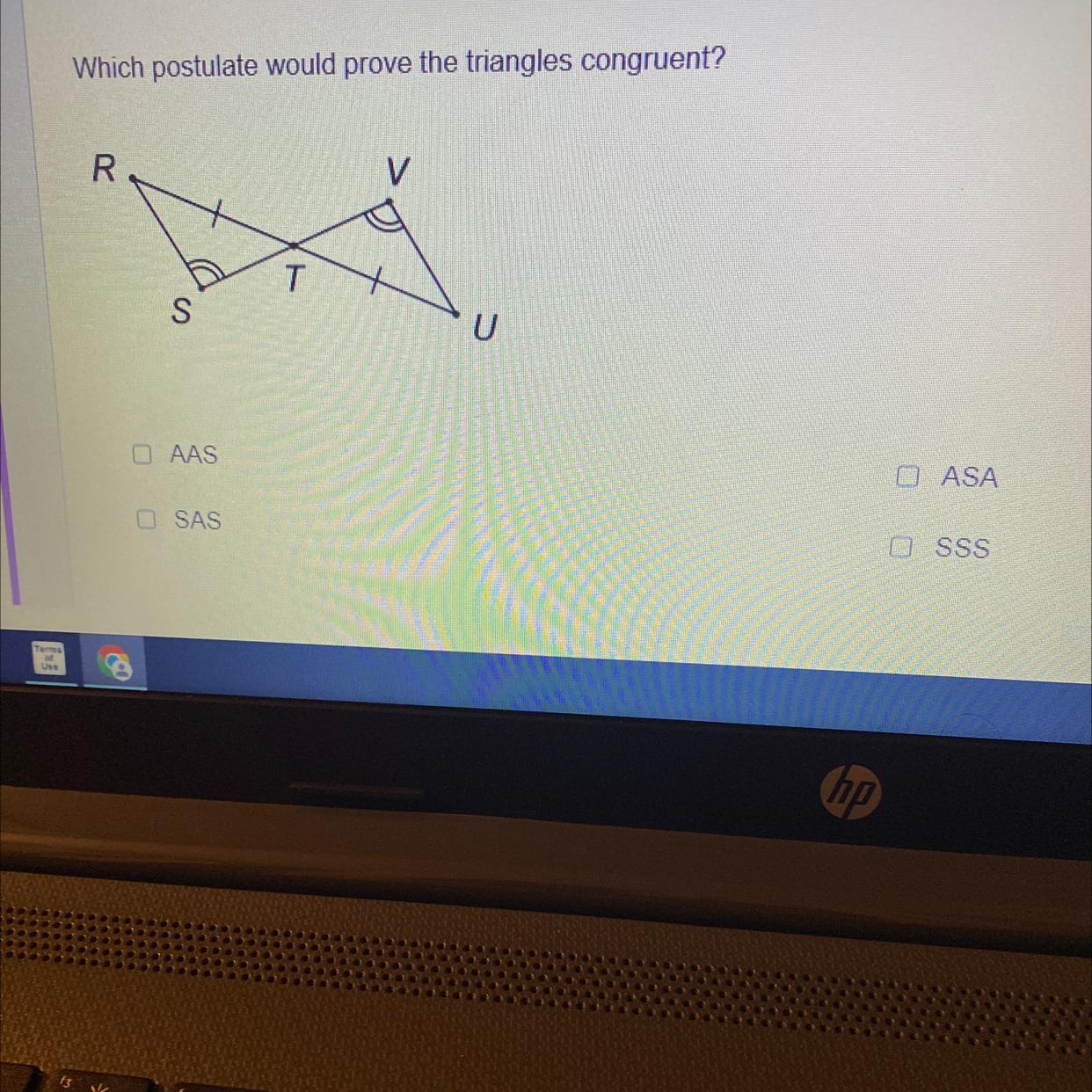
Answer: AAS
Step-by-step explanation:
Two sides are congruent, two angles are congruent, and vertical angles are congruent
Related Questions
A training field is formed by joining a rectangle and two semicircles, as shown below. The rectangle is 81 m long and 60 m wide. Find the area of the training field. Use the value 3.14 for n, and do not round your answer. Be sure to include the correct unit in your answer.
Answers
ANSWER:
EXPLANATION:
Given:
To find:
The area of the training field
We can see that the two semicircles will form a circle with a diameter(d) of 60 m, so the radius(r) of the circle will be;
r = d/2 = 60/2 = 30 m
Given pi as 3.14, we can go ahead and determine the area of the circle as seen below;
[tex]\begin{gathered} Area\text{ of the circle}=\pi r^2 \\ \\ =3.14*30^2 \\ \\ =3.14*900 \\ \\ =2826\text{ m}^2 \end{gathered}[/tex]I need help on a problem
Answers
Those are similar triangles, which means, they are related by a ratio
For example in this case,
7: 10
to find x
10 / 7 = x/3
x= 10*3 /7
x= 30/ 7
x= 4.29
____________
1. Tyra bought a lolli-pop with a diameter of 2 inches. What is the circumference of the lolli-pop to the nearest tenth of an inch? A. 3.9 inches B. 15.7 inches C. 6.3 inches D. 7.9 inches
Answers
A lollypop have a circular shape
Diameter D is the line in a circumference that divides it in half
then calculate directly π• D
to the nearest tenth
π•D = 3.14 x 2 = 6.28
then nearest number is 6.3 , or 6.30. Option C)
Graph the line with the given slope m and y-intercept b
Answers
Answer:
draw a line from the top left to the bottom right going through the centre
Step-by-step explanation:
because m=-1 (gradient)
and b=0 (y intercept)
. . Read the problem and write your answer for each part. Make sure to label each part: , , .jasmine is tracking the growth of a specific bacteria bacteria for a science experiment. She assumes that there are bacteria () in a Perti dish at 12:00 midnight. Jamie observes that the number of bacteria increases by 25 every hour . write an equation that describes the relationship between total number of bacteria () and time () in hours, assuming there are () bacteria in the perti dish at = 0. . if Jamie starts with bacteria in the perti dish, how many bacteria will be present after 6 hours? . if Jamie starts with bacteria in the perti dish, draw a graph that displays the total number of bacteria with respect to time from 12:00 midnight ( = ) to 8:00 am. ( = ). Label each axis and label points on your graph at times = , , , .use the coordinate plane below to draw your graph.
Answers
Part A:
Let:
T(h)= Total Number of bacterias as a function of time
h = Number of hours
B = Initial number of bacterias
Since the number of bacteria increases by 25 every hour, we can defined the equation as:
[tex]T(h)=25h+B[/tex]Part B:
B = 5
h = 6
Evaluate the previous equation for those values:
[tex]\begin{gathered} T(6)=25(6)+5 \\ T(6)=150+5 \\ T(6)=155 \end{gathered}[/tex]there will be 155 baterias after 6 hours
Part C
Let's graph the equation:
[tex]T(h)=25h+5[/tex]4. -X2 + 10 5x + 3 5x+3 6r 4x2 + 2x - 7 I need the perimeter.
Answers
The perimeter is the sum of all sides, therefore:
[tex]\begin{gathered} P=(5x+3)+(4x^2+2x-7)+(-x^2+10)+(5x+3) \\ add_{\text{ }}like_{\text{ }}terms\colon \\ (5x+5x+2x)+(4x^2-x^2)+(3+3+10-7) \\ 3x^2+12x+9 \end{gathered}[/tex][tex]\begin{gathered} 3x^2+12x+9 \\ \frac{1}{3}(3x^2+12x+9)=x^2+4x+3 \end{gathered}[/tex]The factors of 3 that sum to 4 are 3 and 1. So:
[tex]x^2+4x+3=(x+3)(x+1)[/tex]Daniel's family raises honey bees and sells the honey at the farmers' market. To get ready for market day, Daniel fills 24 equal sized jars with honey. He brings a total of 16 cups of honey to sell at the farmers' market.
Use an equation to find the amount of honey each jar holds.
To write a fraction, use a slash ( / ) to separate the numerator and denominator.
Answers
The fraction for the amount of honey each jar holds is 2/3.
What is an equation?A mathematical equation is the statement that illustrates that the variables given. In this case, two or more components are taken into consideration to describe the scenario.
From the information, Daniel fills 24 equal sized jars with honey and he brings a total of 16 cups of honey to sell at the farmers' market.
The amount of honey will be:
= Number of cups / Number of jars
= 16 / 24
= 2/3
Learn more about equations on:
brainly.com/question/2972832
#SPJ1
Determine the value of b.
b3 = 343
b = ±114.3
b = ±7
b = 114.3
b = 7
Answers
Answer:
(d) b = 7
Step-by-step explanation:
You want the solution to b³ = 343.
SolutionThe equation can be written in standard form and factored according to the factoring of the difference of cubes:
b³ -343 = 0
(b -7)(b² +7b +49) = 0
The solutions to this are the values of b that make the factors 0.
b -7 = 0 ⇒ b = 7
b² +7b +49 = 0 ⇒ b = -3.5 ± i√36.75 . . . . . complex solutions
The one real solution to the equation is b = 7.
__
Additional comment
Every cubic has 3 solutions. Here, two of them are complex. When the only terms in the equation are the cubic term and the constant, there will always be only one real root.
<95141404393>
The equation b^3 = 343 has two valid real solutions: b = 7 and b = -7. Both values satisfy the equation and meet the given condition. Option B.
To determine the value of b, we can solve the equation b^3 = 343.
Taking the cube root of both sides, we get:
b = ∛343
The cube root of 343 is 7, since 7 * 7 * 7 = 343. Therefore, one solution to the equation is b = 7.
However, it's important to note that the cube root function has a real and complex solution. In this case, b = 7 is the real solution, but there are two additional complex solutions.
Using complex numbers, we can express the other two solutions as follows:
b = -∛343
b = -7
So the complete set of solutions for b is b = 7, -7.
In summary, the equation b^3 = 343 has two real solutions: b = 7 and b = -7. These solutions satisfy the equation and fulfill the condition. So Option B is correct.
For more question on solutions visit:
https://brainly.com/question/24644930
#SPJ8
Factor 6z^2 + 31z + 18
Answers
Which of the following size measures will form a right triangle
Answers
From the given side lengths, let's find the measures that will form a right triangle.
Apply Pythagorean Theorem:
[tex]a^2+b^2=c^2[/tex]Where:
• a ,and ,b, are lengths of the legs
,• c is the length of the hypotenuse.
Now, let's start from the first measures in option a.
• 48m, 64m, and 85m
[tex]\begin{gathered} 48^2+64^2=85^2 \\ \\ 2304+4096=7225 \\ \\ 6400\ne7225 \end{gathered}[/tex]The measures in option a will NOT form a right triangle since the left side of the equation does not equal the right side.
• 27 yd, 36 yd, 45 yd
[tex]\begin{gathered} 27^2+36^2=45^2 \\ \\ 729+1296=2025 \\ \\ 2025=2025 \end{gathered}[/tex]The measures in option B will form a right triangle because the Equation is true.
ANSWE
3: A Bunch of SystemsSolve each system of equations without graphing and show your reasoning. Then, check yoursolutions,
Answers
Use the elimination method to solve the given system of equations.
To do so, multiply the first equation by -3 so that the coefficient of x in the first equation becomes the additive inverse of the coefficient of x in the second equation:
[tex]\begin{gathered} -3(2x+3y)=-3(16) \\ \Rightarrow-6x-9y=-48 \end{gathered}[/tex]Then, the system is equivalent to:
[tex]\begin{gathered} -6x-9y=-48 \\ 6x-5y=20 \end{gathered}[/tex]Add both equations to eliminate the variable x and to obtain an equation in terms of the variable y only:
[tex]\begin{gathered} (-6x-9y)+(6x-5y)=-48+20 \\ \Rightarrow-6x-9y+6x-5y=-28 \\ \Rightarrow-14y=-28 \\ \Rightarrow y=\frac{-28}{-14} \\ \therefore y=2 \end{gathered}[/tex]Replace y=2 into the first equation to find the value of x:
[tex]\begin{gathered} 2x+3y=16 \\ \Rightarrow2x+3(2)=16 \\ \Rightarrow2x+6=16 \\ \Rightarrow2x=16-6 \\ \Rightarrow2x=10 \\ \Rightarrow x=\frac{10}{2} \\ \therefore x=5 \end{gathered}[/tex]Replace y=2 and x=5 into the second equation to confirm the answer:
[tex]\begin{gathered} 6x-5y=20 \\ \Rightarrow6(5)-5(2)=20 \\ \Rightarrow30-10=20 \\ \Rightarrow20=20 \end{gathered}[/tex]Therefore, the solution to the system of equations is x=5, y=2.
2) (3 pt) Write the function from the table and graph.хf(x)-10004122130.52) f(x) =
Answers
(x - h)^2 = 4p(y - k)
(-1 - 3)^2 = 4p(8 - 0.5)
(-4)^2 = 4p(7.5)
16 = 30p
p = 16/30
p = 8/15
(x - 3)^2 = 16/15(y - 0.5)
15(x^2 - 6x + 9) = 16y - 8
15x^2 - 90x + 135 = 16y - 8
16y = 15x^2 - 90x + 135 + 8
y = 15/16 x^2 - 90/16 x + 143/16
f(x) = 15/16 x^2 - 90/16x + 143/16
You got 84 of 100 questions on the test correct. What percent did you get correct?Answer: 84%100%16%8.4%11/100 is equal to what percent?Answer: 110%10%89%11%3 out of 4 students in your class are girls. What percent of the class are girls?Answer: 3%4%75%25%
Answers
Determine which of the lines are parallel and which of the lines are perpendicular. Select all of the statements that are true.
Line a passes through (-1, -17) and (3, 11).
Line b passes through (0,4) and (7,-5).
Line c passes through (7, 1) and (0, 2).
Line d passes through (-1,-6) and (1, 8).
Answers
Answers:
Line A is parallel to line D.
Line A is perpendicular to line C.
Line C is perpendicular to line D.
=====================================================
Explanation:
Let's use the slope formula to calculate the slope of the line through (-1,-17) and (3,11)
[tex](x_1,y_1) = (-1,-17) \text{ and } (x_2,y_2) = (3,11)\\\\m = \frac{y_{2} - y_{1}}{x_{2} - x_{1}}\\\\m = \frac{11 - (-17)}{3 - (-1)}\\\\m = \frac{11 + 17}{3 + 1}\\\\m = \frac{28}{4}\\\\m = 7\\\\[/tex]
The slope of line A is 7
-------------
Now let's find the slope of line B.
[tex](x_1,y_1) = (0,4) \text{ and } (x_2,y_2) = (7,-5)\\\\m = \frac{y_{2} - y_{1}}{x_{2} - x_{1}}\\\\m = \frac{-5 - 4}{7 - 0}\\\\m = -\frac{9}{7}\\\\[/tex]
-------------
Now onto line C.
[tex](x_1,y_1) = (7,1) \text{ and } (x_2,y_2) = (0,2)\\\\m = \frac{y_{2} - y_{1}}{x_{2} - x_{1}}\\\\m = \frac{2 - 1}{0 - 7}\\\\m = \frac{1}{-7}\\\\m = -\frac{1}{7}\\\\[/tex]
-------------
Lastly we have line D.
[tex](x_1,y_1) = (-1,-6) \text{ and } (x_2,y_2) = (1,8)\\\\m = \frac{y_{2} - y_{1}}{x_{2} - x_{1}}\\\\m = \frac{8 - (-6)}{1 - (-1)}\\\\m = \frac{8 + 6}{1 + 1}\\\\m = \frac{14}{2}\\\\m = 7\\\\[/tex]
------------------------------
Here's a summary of the slopes we found
[tex]\begin{array}{|c|c|} \cline{1-2}\text{Line} & \text{Slope}\\\cline{1-2}\text{A} & 7\\\cline{1-2}\text{B} & -9/7\\\cline{1-2}\text{C} & -1/7\\\cline{1-2}\text{D} & 7\\\cline{1-2}\end{array}[/tex]
Recall that parallel lines have equal slopes, but different y intercepts. This fact makes Line A parallel to line D.
Lines A and C are perpendicular to one another, because the slopes 7 and -1/7 multiply to -1. In other words, -1/7 is the negative reciprocal of 7, and vice versa. These two lines form a 90 degree angle.
Lines C and D are perpendicular for the same reasoning as the previous paragraph.
Line B unfortunately is neither parallel nor perpendicular to any of the other lines mentioned.
You can use a graphing tool like Desmos or GeoGebra to verify these answers.
Suppose you have $14,000 to invest Which of the two rates would yield the larger amount in 2 years 6% compounded monthly or 5.88% compounded continuously?
Answers
We were given a principal to invest ($14,000) in a timespan of 2 years, and we need to choose between applying it on an account that is compounded montlhy at a rate of 6%, and one that is compounded continuously at a rate of 5.88%. To solve this problem, we need to calculate the final amount on both situations, and compare them.
The expression used to calculate the amount compounded monthly is shown below:
[tex]A=P(1+\frac{r}{12})^{12\cdot t}[/tex]Where A is the final amount, P is the invested principal, r is the interest rate and t is the elapsed time.
The expression used to calculate the amount compounded continuously is shown below:
[tex]A=P\cdot e^{t\cdot r}[/tex]Where A is the final amount, P is the invested principal, r is the interest rate, t is the elapsed time, and "e" is the euler's number.
With the two expressions we can calculated the final amount on both situations, this is done below:
[tex]\begin{gathered} A_1=14000\cdot(1+\frac{0.06}{12})^{12\cdot2} \\ A_1=14000\cdot(1+0.005)^{24} \\ A_1=14000\cdot(1.005)^{24} \\ A_1=14000\cdot1.127159 \\ A_1=15780.237 \end{gathered}[/tex][tex]\begin{gathered} A_2=14000\cdot e^{0.0588\cdot2} \\ A_2=14000\cdot e^{0.1176} \\ A_2=14000\cdot1.124794 \\ A_2=15747.12 \end{gathered}[/tex]The first account, that is compounded monthly yields a return of $15780.24, while the second one that is compounded continuously yields a return of $15747.12, therefore the first account is the one that yield the larger amount in 2 years.
A triangle is graphed in this coordinate plane. what is the area of this triangle in square units A.9B.12C.18D.36
Answers
Answer
Option C is correct.
The area of the triangle = 18 square units
Explanation
The area of a triangle is given as
Area of the triangle = ½ × B × H
where
B = Base of the triangle = 6 units (From -3 t
complete by using square x^2 + 4x + 1 = 0
Answers
Given:
The eqution is given as, x^2 + 4x + 1 = 0.
The objective is to solve the equation by compleing the square.
Consider the middle of the equation.
[tex]2\cdot a\cdot b=4x[/tex]Here, the value of a is x. Then, the value of b can be calculated as,
[tex]\begin{gathered} 2(x)\cdot b=4x \\ b=\frac{4x}{2x} \\ b=2 \end{gathered}[/tex]To complete the equation add +b^2 and -b^2 to the equation.
[tex]\begin{gathered} x^2+4x+2^2-2^2+1=0 \\ x^2+4x+2^2-4+1=0 \\ x^2+4x+2^2-3=0 \\ (x+2)^2-3=0 \\ (x+2)^2=3 \end{gathered}[/tex]Take square root on both sides, to solve the value of x,
[tex]\begin{gathered} \sqrt[]{(x+2)^2}=\sqrt[]{3} \\ x+2=\pm\sqrt[]{3} \\ x=\pm\sqrt[]{3}-2 \\ x=+\sqrt[]{3}-2\text{ and -}\sqrt[]{3}-2 \end{gathered}[/tex]Hence, the value of x are +√3-2 and -√3-2.
18. The weights of four puppies are shown in pounds. 9.5 9 9.125 9 Which list shows these weights in order from greatest to least F. 99.5 9 9.125 w 9.5 9 9.125 9.125 9 9.5 9 + J. 9 9 9.5 9.125
Answers
The correct list is
[tex]9\frac{3}{4},\text{ 9.5, 9}\frac{3}{8},9.125[/tex]This is option F
Help 50 points (show ur work)
Answers
1. The value of 34% of 850 is 289.
3. The amount that Kepley paid for the tool is $120.
How to calculate the value?From the information, we want to calculate 34% of 850. This will be calculated thus:
= 34% ×850
= 34/100 × 850
= 0.34 × 850
= 289
The amount paid for the tool will be:
= Price or tool - Discount
= $200 - (40% × $200)
= $200 - $80
= $120
Learn more about percentages on:
brainly.com/question/24304697
#SPJ1
The answer for the bottom question need a fast quick answer
Answers
Area of a circle is
[tex]A=\pi\cdot r^2[/tex][tex]\begin{gathered} d=2r \\ d=22 \\ r=\frac{22}{2} \\ r=11 \end{gathered}[/tex][tex]\begin{gathered} A=\pi(11)^2 \\ A=380.133ft^2 \end{gathered}[/tex]The area of the garder is 380.13 square footsIf a square foot cost $ 1.25
[tex]\begin{gathered} 1ft^2\to1.25\text{dollars} \\ 380.13ft^2\to x \\ x=\frac{380.13ft^2\cdot(1.25dollar)}{1ft^2} \\ x=475.16\text{dollar} \end{gathered}[/tex]To cover the garden they need to buy $475 in mulchLeah invested $400 in an account paying an interest rate of 1 1/2%compounded annually. Lauren invested $400 in an account paying aninterest rate of 0 7/8% compounded monthly. To the nearest hundredth of ayear, how much longer would it take for Lauren's money to triple than forLeah's money to triple?
Answers
Leah investment is:
[tex]M_{\text{Leah}}=400_{}\cdot1.5^y[/tex]Where M is the ammount of money that she has, and y the number of years.
We want to know the number of years that must elapse for her investment to triple, so we want to know the value of y such that:
[tex]\begin{gathered} 3\cdot400=400\cdot(1+\frac{1.5}{100})^y \\ 3=(1.015)^y \\ \ln 3=y\cdot\ln (1.015) \\ y=\frac{\ln (3)}{\ln (1.015)}\cong73.788\cong73.79 \end{gathered}[/tex]It will take 73.79 years to triple her investment.
Lauren investment is:
[tex]M_{\text{Lauren}}=400\cdot(1+\frac{7}{8}\cdot\frac{1}{100})^m=400\cdot(1.00875)^{\frac{y}{12}}[/tex]Where M is the ammount of money that she has, and m the number of months, and y is the number of years.
We want to know the number of years that must elapse for her investment to triple, so we want to know the value of y such that:
[tex]\begin{gathered} 3\cdot400=400\cdot(1.00875)^{\frac{y}{12}} \\ 3=(1.00875)^{\frac{y}{12}} \\ \ln 3=\frac{y}{12}\ln (1.00875) \\ y=12\cdot\frac{\ln 3}{\ln (1.00875)} \\ y=1513.25 \end{gathered}[/tex]Can someone explain how I would know the difference between a 2:7 ratio and 7:2 ratio when a point partitions the line? Thank you!
Answers
Solution
For this case we can do the following:
We can understand 7/2 as the reciprocal of 2/7 and we can create the following diagram
Calculate the degree of the angles in the triangles below.
Answers
the sum of the internal angles of a triangle is equal to 180, then
[tex]\begin{gathered} 2x+7+5x+12=180 \\ 7x+19=180 \\ 7x+19-19=180-19 \\ 7x=161 \\ \frac{7x}{7}=\frac{161}{7} \\ x=23 \end{gathered}[/tex]so
answer:
angle 1 = 2x + 7 = 2(23) + 7 = 46 + 7 = 53°
angle 2 = 5x = 5(23) = 115°
angle 3 = 12°
Tye mapping diagram below matches the points on the graph next to it.find the value of X
Answers
Given,
The coordinates of the points shown in the graph is:
[tex](-6,2),\text{ \lparen6,2\rparen, \lparen6,1\rparen and \lparen3,1\rparen}[/tex]Here, the given mapping is:
[tex](-6,2),\text{ \lparen6,2\rparen, \lparen x,1\rparen and \lparen3,1\rparen}[/tex]On comparing the coordinates then x = 6.
Hence, the value of x is 6.
Q.Using triangle congruency theorems, justify why the triangles are similar.
Answers
4a)
Looking at the figure,
AD and BE are parallel lines
AE and BD are transversals
Thus,
angle DAE is congruent to angle BEA because they are alternate angles(They lie in similar positions but on alternate sides of the transversal
Also,
angle ADB is congruent to angle EBD because they are alternate angles(They lie in similar positions but on alternate sides of the transversal.
Angle ACD is congruent to angle BCE
Recall, 2 triangles are similar if at least, 2 of their corresponding angles are congruent. In this case, 3 corresponding triangles are congruent. Thus,
triangle ADC is similar to triangle EBC by AA(angle angle) postulate
4b) If 2 triangles are similar, it means that the ratio of their corresponding sides is equal. Thus,
AD/EB = DC/BC = AC/ CE
From the information given,
AD = 2
AC = 4
EB = 5
Thus,
2/5 = 4/CE
By cross multiplying,
2 * CE = 5 * 4
2CE = 20
CE = 20/2
CE = 10
Cecilia is making a punch that uses 3 quarts of fruit juice for every quart of citrus soda. How many quarts of each does she need to make 16 quarts of punch?
Answers
The number of quarts of each constituent she needs to produce 16 quarts of punch is; 12 quarts of fruit juice and 4 quarts of citrus soda.
What quantity of each constituent is needed to produce 16 quarts of punch?It follows from the task content that the quantity of each constituent that is required to make 16 quarts of punch are to be determined.
Since it follows that 3 quarts of fruit juice are required to mix with every quart of citrus soda, it follows that the amount of punch made in this scenario is; 4 quarts of punch.
Hence, since there are 4 partitions of 4 quarts punch is 16 quarts punch, the amount of.each constituent needed by proportion are as follows;
3 quarts of fruit juice × 4 = 12 quarts of fruit juice.1 quarts of fruit juice × 4 = 4 quarts of citrus soda.Read more on proportion;
https://brainly.com/question/18437927
#SPJ1
a digital music player is marked down from its list price of $249.99 to a sale price of $194.99. What is the discount rate?
Answers
The discount rate of the digital player is 22%
How to determine the digital player's discount rate?From the question, we have the given parameters:
List price = $249.99
Sales price = $194.99
Start by calculating the change in the price.
This is calculated as follows
Change = List price - Sales price
So, we have
Change = $249.99 - $194.99
Evaluate the difference
Change = $55
The discount rate of the digital player is then calculated as
Discount = Change/List price x 100%
This gives
Discount = 55/249.99 x 100%
Evaluate
Discount = 22%
Hence, the discount rate is 22%
Read more about discount rate at
https://brainly.com/question/7459025
#SPJ1
The probability of a certain brand of battery going dead within 15 hours is 1/3. Noah has a toy that requires 4 of these batteries. He wants to simulate the situation to estimate the probability that at least one battery will die before 15 hours are up. 1. Noah will simulate the situation by putting marbles in a bag. Drawing one marble from the bag will represent the outcome of one of the batteries in the toy after 15 hours. Red marbles represent a battery that dies before 15 hours are up, and green marbles represent a battery that lasts longer. How many marbles of each color should he put in the bag? Explain your reasoning. *
Answers
a.
Noah put marbles on a bag, each color marble represents that the battery of the toy died before 15 hours (red marbles) or that the battery lasted after 15 hours (green marbles)
There are 4 batteries on the toy, for each battery there are two possible outcomes, each one represented by a red and green marble.
So, for each battery, he has to put two marbles. There will be 4 red marbles and 4 green marbles in the bag.
b.
To estimate the probability that at least one battery will die within 15 hours, you have to calculate the expected value.
a) Twice the difference of a number c and forty.b) Four times the sum of a number f and fifty.
Answers
a) We have a number X that is twice the difference of a number c and 40.
We can write this as:
[tex]X=2(c-40)[/tex]b) Four times the sum of a number f and fifty.
Then, X is:
[tex]X=4(f+50)[/tex]Tickets numbered 1 - 10 are drawn at random and placed back in the pile. Find the probability that at least one ticketnumbered with a 6 is drawn if there are 4 drawings that occur. Round your answer to two decimal places.
Answers
The probability of a 6 being drawn in one pick is
[tex]\frac{1}{10}[/tex]For 4 drawings, the probability would be
[tex]\frac{1}{10}+\frac{1}{10}+\frac{1}{10}+\frac{1}{10}=\frac{4}{10}=\frac{2}{5}=0.40[/tex]